|

|
Lines, angles, figures,
Puzzles waiting to be solved,
Geometry fun.
|
|

|
Open Geometry Problems
Problems
Ten Geometry Problems
Visual Index
Parallel lines
Perpendicular lines
Collinear Points
Triangle
Metric Relations
Quadrilateral
Triangle & Squares
Polygons
Pentagons
Hexagons
Heptagons
Octagons
Circle
Triangle
Centers
Congruence
Similarity, Ratios, Proportions |
|
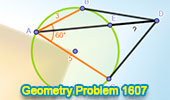 |
Geometry Problem 1607: Circle, Chords, Tangents, 60 Degree Angle |
|
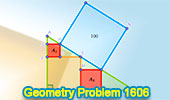 |
Geometry Problem 1606: Nested Squares Area Challenge |
|
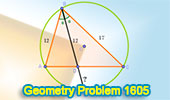 |
Geometry Problem 1605: Triangle, Angle Bisector, and Circumcircle |
|
 |
Geometry Problem 1604: Explore this elegant problem involving two circles, a midpoint, a tangent, and an unknown segment |
|
 |
Geometry Problem 1603: One square. One circle. One secant from point C. A square inside a circle reveals a geometric mystery. Are you ready to solve it? |
|
 |
Geometry Problem 1602: Find the Distance from the Semicircle’s Center to the Incenter |
|
 |
Dynamic Geometry Problem 1601: Rectangular Property of Cyclic Orthodiagonal Quadrilaterals |
|
 |
Geometry Problem 1600: When Cevians Cross: Find the Missing Area: A STEM Geometry Challenge |
|
 |
Geometry Problem 1599: Four Equal Squares, One Right Triangle: What’s the Ratio?: A STEM Geometry Challenge |
|
 |
Geometry Problem 1598: Inradius Ratio with Median BM in a Right-Angled Triangle: A STEM Geometry Challenge |
|
 |
Geometry Problem 1597: Circle-Triangle Intersection Problem: A STEM Geometry Challenge |
|
 |
Geometry Problem 1596: Triangle Projections: Unraveling the DE Projection with Equal Angles!: A STEM Geometry Challenge |
|
 |
Geometry Problem 1595: The Rectangle's Area and Its Relationship with Three Strategic Triangles – A STEM Perspective |
|
 |
Geometry Problem 1594: Intersecting Squares & Hidden Lengths – A STEM Perspective |
|
 |
Geometry Problem 1593: Find BF in Right Triangle ABC – A STEM Perspective |
|
 |
Geometry Problem 1592: A Semicircle Challenge for Mental Clarity Involving Radii, Perpendiculars, Midpoint |
|
 |
Geometry Problem 1591: Unravel Quadrilateral ABCD with Three Congruent Sides and Proportional Angles to Find Angle D. |
|
 |
Geometry Problem 1590: Midpoints, Incircles,
Areas, and the Art of Balance. |
|
 |
Geometry Problem 1589: Unlock the Mystery: Prove the Ratio of BD to OD is
Square Root of Two in a 90° Circular Sector!. |
|
 |
Geometry Problem 1588: Unlocking the Tangent: Prove the Fifth of the
Radius in a Sector with Semicircle. |
|
 |
Geometry Problem 1587: Uncover the Elegance of Triangle BGH's Area in
Trapezoid ABCD. |
|
 |
Geometry Problem 1586: Unveiling a Unique Area Relationship in Three
Equilateral Triangles |
|
 |
Geometry Problem 1585: Discover how a triangle's incircle reveals the
secret of harmonious convergence |
|
 |
Geometry Problem 1584: Proving a Ratio Involving Incircle and Angle
Bisector
Unveiling a Potentially Unexplored Geometric Relationshp. |
|
 |
Geometry Problem 1583: Prove Lines CK and BL are Parallel. |
|
 |
Geometry Problem 1582: Prove That Angles AFD and AEF Are Equal in This
Secant Problem. |
|
 |
Geometry Problem 1581: Prove the Angle Bisection in a Cyclic
Quadrilateral. |
|
 |
Geometry Problem 1580: 60-Degree Triangle Challenge: Uncover AD Using
Excenter and Circumcircle Clues. |
|
 |
Geometry Problem 1579: Involving perpendicular diameters, tangents, and
triangle areas. |
|
 |
Geometry Problem 1578: Find the Area of a Bicentric Quadrilateral with
Perpendicular Extensions of Opposite Sides!. |
|
 |
Geometry Problem 1577: Prove that in triangle ABC, segment AB equals the
sum of segments BD and CD, with given angles and congruent segments. |
|
 |
Geometry Problem 1576: Congruency of Segments in Triangle ABC with Angles
30 and 20 Degrees and an Interior Cevian |
|
 |
Geometry Problem 1575: Prove an Angle Bisector in a Triangle Involving an
Altitude, Midpoint, and Excircle |
|
 |
Geometry Problem 1574: Triangle with Three Circles through a Point and the
Concyclicity of Six Intersection Points |
|
 |
Geometry Problem 1573: Two circles are externally tangent at point C.
Given specific secants and tangents, find the angle at point K |
|
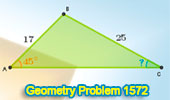 |
Geometry Problem 1572: Calculate Angle C with Geometric Methods Using
Sides AB=17, BC=25, and Angle A=45 Degrees |
|
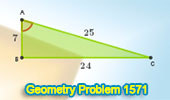 |
Geometry Problem 1571: Prove that the intermediate angle in a Pythagorean
Triple 7-24-25 measures 74 degrees. |
|
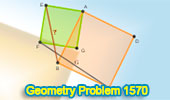 |
Geometry Problem 1570: Calculating BE in Overlapping Squares: A High
School and College Geometry Challenge. |
|
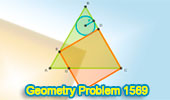 |
Geometry Problem 1569: Prove a Relationship: EG Equals the Incircle
Diameter in Triangle ABC with Square CDEF. |
|
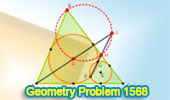 |
Geometry Problem 1568: Concyclicity of Points B, D , H, J in a Triangle
ABC with an Incircle and a Tangent Circle. |
|
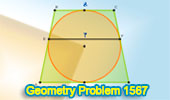 |
Geometry Problem 1567: Finding Tangent Distances in a Circumscribed
Isosceles Trapezoid. |
|
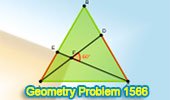 |
Geometry Problem 1566: Demonstrate AC Equals AE + CD in an Equilateral
Triangle ABC Involving Cevians and 60 Degrees.. |
|
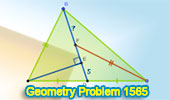 |
Geometry Problem 1565: Find the Length of BF in Triangle ABC Involving
Median, Perpendicular, Midpoint, and Congruence. |
|
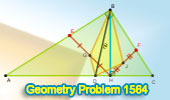 |
Geometry Problem 1564: Find the Area of Quadrilateral BGDJ in a Right
Triangle involving the Altitude, Angle bisectors, and Midpoints. |
|
 |
Geometry Problem 1563: Perpendicularity in a Right Triangle involving the
Altitude, Angle bisectors, and Midpoints. |
|
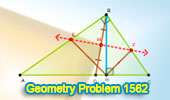 |
Geometry Problem 1562: Proof of Collinearity in a Right-angled Triangle
involving the Altitude, Angle bisectors, and Midpoint. |
|
 |
Geometry Problem 1561: Triangle ABC, Circumcenter O, Orthocenter H,
Parallel Line, and Angle Secrets. |
|
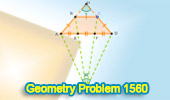 |
Geometry Problem 1560: Trapezoid ABCD: Unlocking Angle Secrets at G and H. |
|
 |
Geometry Problem 1559: Proving BC Bisects Segment DE. This geometry
problem challenges students at high school and college levels. |
|
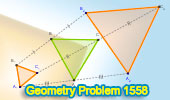 |
Geometry Problem 1558: The midpoints of segments connecting corresponding
vertices of equilateral triangles form an equilateral triangle. |
|
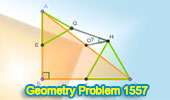 |
Geometry Problem 1557: Calculate the Angle DHG in Right Triangle ABC. The
problem involves: equilateral, isosceles, midpoint and congruence |
|
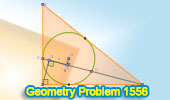 |
Geometry Problem 1556: Right Triangle ABC and Inscribed Circle. The
problem involves circle, chords, tangent, perpendicular lines, and
congruence |
|
 |
Geometry Problem 1555: Find Length of DE. The problem involves chords,
tangent, circles, and intersections of line |
|
 |
Geometry Problem 1554: Finding the Length of Side AB in Triangle ABC |
|
 |
Geometry Problem 1553: Solving for OC in Triangle ABC with Unique Angle
Bisectors |
|
 |
Geometry Problem 1552: Exploring Angle C in Triangle ABC with Given Angle
A and Side Lengths |
|
 |
Geometry Problem 1551: Unraveling Angle Relations in Cyclic
Quadrilaterals: Solving for Angle GEJ: A High School Level Investigation |
|
 |
Geometry Problem 1550: Solving for Segment BD: An Angle Puzzle in Right
Triangle ABC: A High School Level Investigation |
|
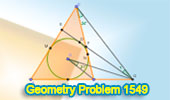 |
Geometry Problem 1549: Unraveling the Geometric Mystery: Calculating Angle
BGE with the Incircle and Tangent in Triangle ABC |
|
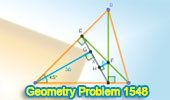 |
Geometry Problem 1548: Exploring Segment DE Length in Triangle ABC with a
45-Degree Angle and Intersecting Altitudes |
|
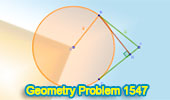 |
Geometry Problem 1547: Tangents' Dance: Exploring B-to-AC Distance in a
Circle's Grasp |
|
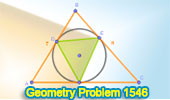 |
Geometry Problem 1546: Discover the Hidden Geometry: Calculate Area of
Contact Triangle DEF in Triangle ABC |
|
 |
Geometry Problem 1545: Unlock the Geometric Mystery: Calculate the Area of
Triangle ABC with Inscribed Circle and Excenters! |
|
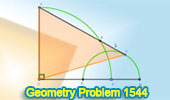 |
Geometry Problem 1544: Challenge: Calculate the Area of a Triangle with
Given Arc and Semicircle Intersections. |
|
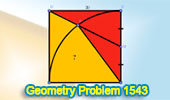 |
Geometry Problem 1543: Calculating the Area of Quadrilateral ABED in a
Square with a Side Length of 20 and an Intersecting Arc. |
|
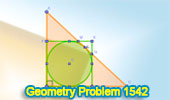 |
Geometry Problem 1542: Unraveling a Geometric Puzzle with a Circumscribed
Right Triangle and Square to the Same Circle for School and College. |
|
 |
Geometry Problem 1541 Challenge: Unveiling BG Length in an Inscribed
Quadrilateral with Harmonic Quaternary Insight for Academic Pursuit. |
|
 |
Geometry Problem 1540: Solving for the Length of Chord in a Circle:
Analyzing Intersections and Given Values for Academic Pursuits. |
|
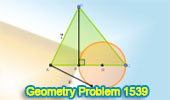 |
Geometry Problem 1539 Demystified: Unraveling the Lengths in an Isosceles
Triangle with Altitude and Tangent Secrets!. |
|
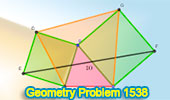 |
Geometry Problem 1538: Unlocking the Secrets of Triangular Geometry: Solve
for the Area of a Quadrilateral Using External Squares and a Segment
Length. |
|
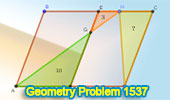 |
Geometry Problem 1537 Challenge: Can You Solve for the Missing Area in a
Parallelogram using Midpoints and Intersection Points?. |
|
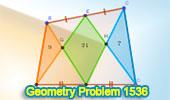 |
Geometry Problem 1536: Discover the Power of Midpoints: Solving for
Missing Areas in Quadrilaterals. |
|
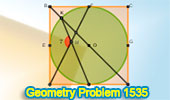 |
Geometry Problem 1535: Crack the Code: Inscribed Circle in Square - Angle
Challenge! Solve the Mystery. |
|
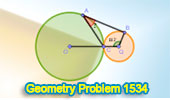 |
Geometry Problem 1534: High School Brainteaser: Tangent Circles, Common
External Tangent, and Angle Conundrums!.
. |
|
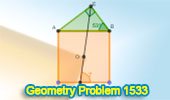 |
Geometry Problem 1533: Discovering Relationships between Angles and Lines
in an Exterior Right Triangle of a Square - A High School Challenge.
. |
|
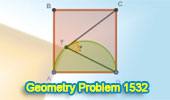 |
Geometry Problem 1532: Crack the Code of Geometry Problem 1532: How to
Find the Angle in a Square with a Tangent Semicircle! - A High School
Challenge.
. |
|
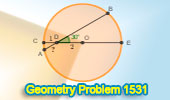 |
Geometry Problem 1531: Discover How to Calculate the Length of a Chord in
a Circle with Diameter Intersection and an Angle between the Diameter and
Chord - A High School Challenge.
. |
|
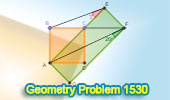 |
Geometry Problem 1530: Unlock the Secrets of Geometric Angles: Calculate
the Measurement of an Angle in a Square and Rectangle Figure Today! - A
High School Challenge.
. |
|
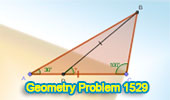 |
Geometry Problem 1529: Unlock the Mystery of Triangles: Solving for the
Missing Angle with 100-50-30 Degree Angles and Cevian Lengths - A High
School Challenge.
. |
|
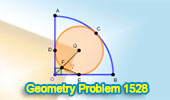 |
Geometry Problem 1528: Cracking the Circle Code: Unveiling the Tangent and
Angle of an Inscribed Circle within a 90-Degree Circular Sector.
. |
|
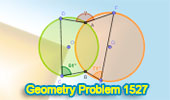 |
Geometry Problem 1527: Discovering the Hidden Angle: Solving the Puzzle of
Two Intersecting Circles.
. |
|
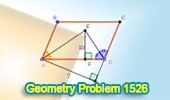 |
Geometry Problem 1526: Mastering Geometry Problem-Solving: Discover the
Distance Between Two Sides in a Parallelogram Using Bisectors and Distance
Measures.
. |
|
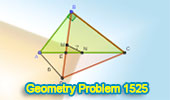 |
Geometry Problem 1525 and a Thematic Poem.
Unveiling the Secrets of an Equilateral Triangle in Right Triangle
Geometry: Finding the Midpoint Distance between Segments. |
|
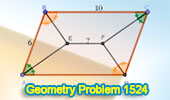 |
Geometry Problem 1524 and a Thematic Poem.
Unlock the Mystery of Parallelograms: Discover the Length of Segment
between the Intersecting Angle Bisectors. |
|
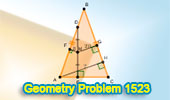 |
Geometry Problem 1523 and a Thematic Poem.
Discover How to Calculate the Length of the Altitude in an Isosceles
Triangle - Get Expert Geometry Tips Now! . |
|
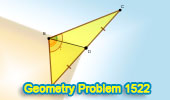 |
Geometry Problem 1522 and a Thematic Poem.
Unlocking the Angle Measure of a Triangle with Median and Doubled Side
Lengths. |
|
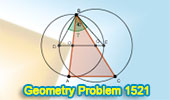 |
Geometry Problem 1521 and a Thematic Poem.
Unlock the Secret to Finding the Measure of an Angle in a Triangle with
Two Sides as Diameters of Circles. |
|
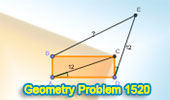 |
Geometry Problem 1520 and a Thematic Poem.
Discovering Distances in a Rectangle with an Exterior Point: A Geometry
Challenge. |
|
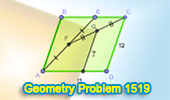 |
Geometry Problem 1519 and a Thematic Poem.
Discover the Length of a Segment in a Parallelogram using Midpoints and
Parallel Lines. |
|
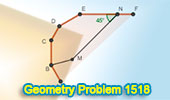 |
Geometry Problem 1518 and a Thematic Poem.
Boost Your Geometry Skills: Solve for the Number of Sides in an
Equiangular Polygon with an Interior Point and Bisected Angle. |
|
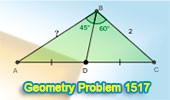 |
Geometry Problem 1517 and a Thematic Poem.
Unlocking Triangle Side Length: Solving with a Median and Two Angles.
Difficulty Level: High School. |
|
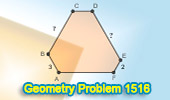 |
Geometry Problem 1516 and a Thematic Poem.
Finding the Length of a Side in an Equiangular Hexagon with Given Three
Side Lengths. Difficulty Level: High School. |
|
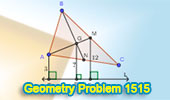 |
Geometry Problem 1515 and a Thematic Poem.
Mastering Triangle Distance Calculation: Find the Distance from the
Intersection of Medians to an Exterior Line. Difficulty Level: High
School. |
|
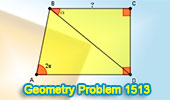 |
Geometry Problem 1513 and a Thematic Poem.
Solving the base in a Right Trapezoid with Double Angle and Sum of Two
Sides. Difficulty Level: High School. |
|
 |
Geometry Problem 1512 and a Thematic Poem.
Finding the Length of a Segment in a Triangle with a Median and a Cevian
with Given Ratio. Difficulty Level: High School. |
|
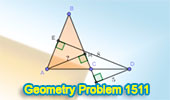 |
Geometry Problem 1511 and a Thematic Poem.
Finding the Altitude of an Isosceles Triangle Using Distances from a Point
on the Extension of the Base. Difficulty Level: High School. |
|
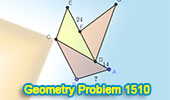 |
Geometry Problem 1510 and a Thematic Poem.
Congruence of Triangles, Perimeter, Measurement. Difficulty Level: High
School. |
|
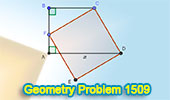 |
Geometry Problem 1509 and a Thematic Poem.
Congruence of Triangles in a Trapezoid and a Square, Measurement.
Difficulty Level: High School. |
|
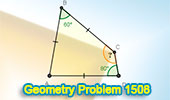 |
Geometry Problem 1508.
Understanding Triangle Geometry: Isosceles, Equilateral, Quadrilateral,
Angular. Difficulty Level: High School. |
|
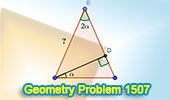 |
Geometry Problem 1507.
Understanding Triangle Geometry: Double Angles, Altitudes, and
Measurements. Difficulty Level: High School. |
|
 |
Geometry Problem 1506.
Parallel Rays, Angles, Corresponding, Alternate. |
|
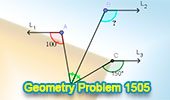 |
Geometry Problem 1505.
Parallel Rays, Angles, and Bisector, Corresponding, Alternate, Conjugate. |
|
 |
Geometry Problem 1504 and Poem.
Squares, Equilateral Triangle, Parallel, Angle. |
|
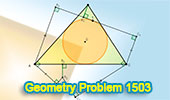 |
Geometry Problem 1503.
Triangle, Incircle, Tangent, Congruence, Perpendicular. |
|
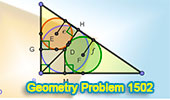 |
Geometry Problem 1502.
Right Triangle, Incircle, Inradius, Geometric Mean of 2 Inradii, Angle
Bisector, Perpendicular, Tangential Quadrilateral. |
|
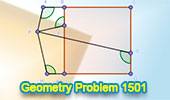 |
Geometry Problem 1501.
Square, Exterior Point, Congruent Angles, Segment, Measurement. |
|
 |
Geometry Problem 1500.
Circle, Perpendicular, Tangent, Secant, Cyclic Quadrilateral, Parallel,
Similarity, Measurement. |
|
 |
Dynamic Geometry 1450.
Ortholine, Steiner Line, Complete Quadrilateral, Collineal Orthocenters.
Step-by-step illustration using GeoGebra. |
|
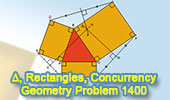 |
Geometry Problem 1400.
Triangle with three rectangles on the sides,
Perpendicular Bisectors, Concurrency. |
|
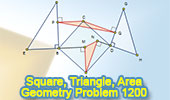 |
Geometry Problem 1200
Square, Right Triangle, Perpendicular, 90 Degrees, Equal Areas. |
|
 |
Geometry Problem 900
Intersecting Circles, Common External Tangent, Secant,
Angle, Congruence.
GeoGebra, HTML5 Animation for Tablets.
Solution. |
|
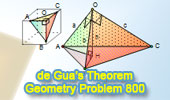 |
Geometry Problem 800
de Gua's Theorem, Pythagorean theorem in 3-D, Tetrahedron, Cubic Vertex,
Triangular Pyramid, Apex, Height, Right Triangle Area, Base Area,
Projected Area.
Solution. |
|
 |
Geometry Problem 700.
Equilateral Triangle, Circle, Circular Segment, Midpoint of
a side, Metric Relations.
Solution. |
|
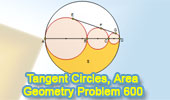 |
Geometry Problem 600
Circle, Area, Tangent, Semicircle, Tangency Point.
Solution. |
|
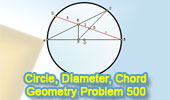 |
Geometry Problem 500.
Circle, Diameter, Chord, Perpendicular, Measure.
Solution. |
|
 |
Geometry Problem 400.
Triangle, Angle bisector, Circumcircle, Perpendicular, Congruence.
Solution. |
|
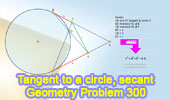 |
Geometry Problem 300.
Tangents to a circle, Secants, Square.
Solution. |
|
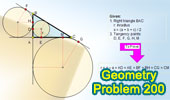 |
Geometry Problem 200.
Right Triangle, Incircle, Excircles, Points of Tangency, Inradius.
Solution. |
|
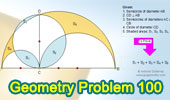 |
Geometry Problem 100.
Circle Area, Archimedes' Book of Lemmas.
Solution. |
|
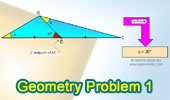 |
Geometry
Problem 1.
Triangle, median, angles, 45 degrees.
Solution |
|
Go to Page: Previous | 1 |
2 |
3 |
4 |
5 |
6 |
7 |
8 |
9 |
10 |
11 |
12 |
13 |
14 |
15 |
Next |