Challenge Your Geometry Skills with Problem 1456: Altitudes, Orthic Triangle, Circumcircle, Parallel, Similarity, and Area

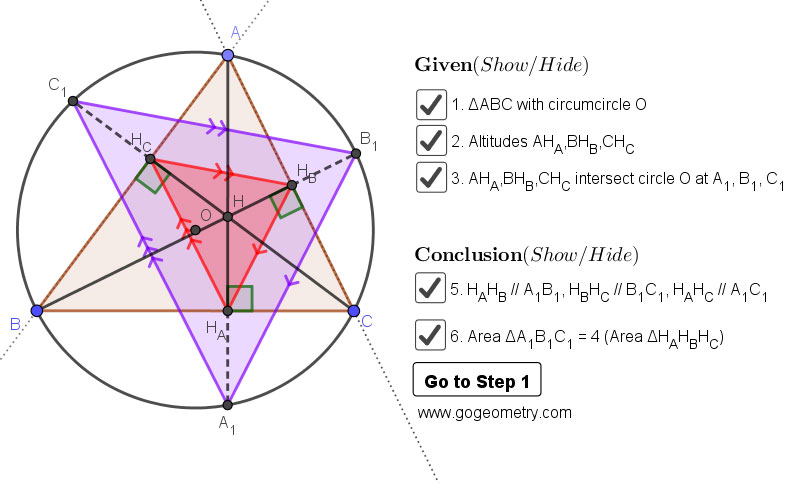
Let AH_A, BH_B, CH_C be the altitudes of triangle ABC. The extensions of AH_A, BH_B, and CH_C intersect the circumcircle O at A₁, B₁, and C_1,respectively. Prove that (1) H_AH_B // A₁B₁, H_BH_C // B₁C₁, and H_AH_C // A₁C₁; (2) the area of triangle A₁B₁C₁ is 4 times the area of triangle H_AH_BH_C.

See solution below

Static Diagram of problem 1456

Dynamic Geometry 1456: Altitude, Orthic Triangle, Circumcircle, Similarity, Area. Using GeoGebra

Poster of the problem 1456 using iPad Apps

Poster of Problem 1456, Altitude, Orthic Triangle, Circumcircle, Similarity, Area. Using iPad

Search gogeometry.com

Classroom Resource:
Interactive step-by-step animation using GeoGebra

This step-by-step interactive illustration was created with GeoGebra.

To explore (show / hide): click/tap a check box.
To stop/play the animation: click/tap the icon in the lower left corner.
To go to first step: click/tap the "Go to step 1" button.
To manipulate the interactive figure: click/tap and drag the blue points or figures.

GeoGebra is free and multi-platform dynamic mathematics software for all levels of education that joins geometry, algebra, tables, graphing, statistics and calculus application, intended for teachers and students. Many parts of GeoGebra have been ported to HTML5.

Recent Additions

Geometry Problems
Open Problems
Visual Index
Ten problems: 1411-1420
All Problems
Triangle
Circle
Circumcircle
Triangle Centers
Altitude
Orthocenter
Perpendicular lines
Parallel lines
Orthic Triangle
Similarity, Ratios, Proportions
Triangle Area
Dynamic Geometry
GeoGebra
HTML5 and Dynamic Geometry
iPad Apps
View or Post a solution