Geometry Problem 1522: Unlocking the Angle Measure of a Triangle with Median and Doubled Side Lengths. Difficulty Level: High School.

Consider a triangle ABC in which BD is the median, angle ABD is twice angle DBC, and BC is twice the length of BD. Find the measure of angle ABC.

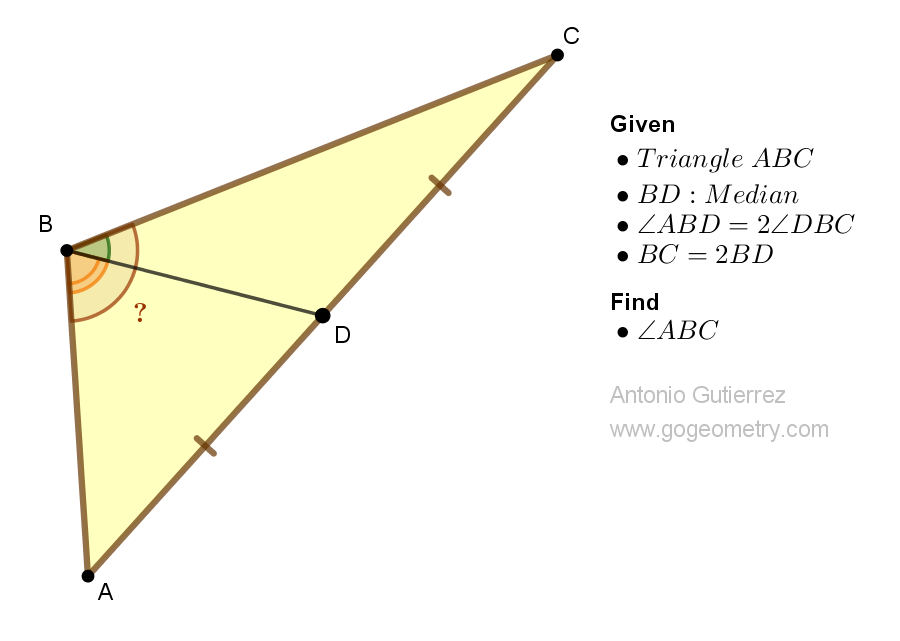
Geometry Problem 1521: UUnlocking the Angle Measure of a Triangle with Median and Doubled Side Lengths. Difficulty Level: High School.

View or post a solution

Definitions and Suggestions

Concept Definition Theorem
Triangle A polygon with three sides and three angles. The sum of the angles in a triangle is 180 degrees.
Median A line segment that connects a vertex of a triangle to the midpoint of the opposite side. In a triangle, the medians from any two vertices intersect at a point that is two-thirds of the way along the median from the vertex to the midpoint of the opposite side.
Midpoint The point that divides a line segment into two equal parts. The midpoint theorem for triangles, also known as the mid-segment theorem, states that the segment connecting the midpoints of two sides of a triangle is parallel to the third side and half of its length.
Parallel lines Two lines in a plane that do not intersect. If two parallel lines are cut by a transversal, then the alternate interior angles are congruent, the corresponding angles are congruent, and the consecutive interior angles are supplementary.
 

Discover Even More: See Also...

Problem 1523

Geometry Problem 1523: Discover How to Calculate the Length of the Altitude in an Isosceles Triangle - Get Expert Geometry Tips Now!

Problem 1521

Geometry Problem 1521: Unlock the Secret to Finding the Measure of an Angle in a Triangle with Two Sides as Diameters of Circles. Difficulty Level: High School.

Problem 1520

Geometry Problem 1520: Discovering Distances in a Rectangle with an Exterior Point: A Geometry Challenge. Difficulty Level: High School.

Problem 1519

Geometry Problem 1519: Discover the Length of a Segment in a Parallelogram using Midpoints and Parallel Lines. Difficulty Level: High School.

Problem 1518

Geometry Problem 1518: Boost Your Geometry Skills: Solve for the Number of Sides in an Equiangular Polygon with an Interior Point and Bisected Angle. Difficulty Level: High School.

Problem 1517

Geometry Problem 1517: Unlocking Triangle Side Length: Solving with a Median and Two Angles. Difficulty Level: High School.

Thematic Poem:
Problem 1522: Unveiling the Secrets of a Triangle: A Geometry Spree

In a triangle bold and true,
BD the median, divides it in two.
ABD angle twice DBC,
A fact that will set your geometry free.

BC doubled, BD too,
A puzzle that's challenging, but worth the view.
The angle measure of ABC,
Is what we seek, for our geometry spree.

Unlock the secrets of this shape,
Find the measure that's hidden away.
Let your mind and compass guide,
And the angle measure will no longer hide.

So measure and calculate with ease,
And discover the beauty that geometry sees.
The angles of a triangle, we unveil,
A joyous discovery, we now hail. 

If you're interested in finding more poems with a focus on geometry, you may enjoy this collection: More geometry thematic poems.

Recent Additions

Geometry Problems
Open Problems
Visual Index
All Problems
Triangle
Median
Midpoint
Parallel lines
View or Post a solution 

Search our site with Google: