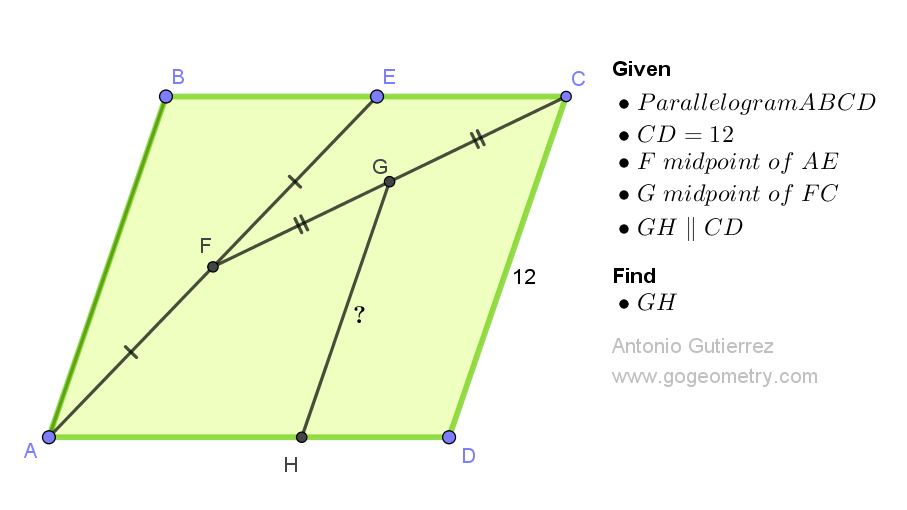
Geometry Problem 1519: Discover the Length of a Segment in a Parallelogram using Midpoints and Parallel Lines. Difficulty Level: High School.

Consider a parallelogram ABCD where CD has a length of 12 units. Let E be a point on the line segment BC, and let F be the midpoint of the line segment AE. Also, let G be the midpoint of the line segment FC. A line segment GH is drawn parallel to CD through G, such that H lies on the line segment AD. Find the length of GH.

Geometry Problem 1519: Discover the Length of a Segment in a Parallelogram using Midpoints and Parallel Lines. Difficulty Level: High School.

Definitions and Suggestions

Theorem Description
Parallelogram A parallelogram is a quadrilateral with both pairs of opposite sides parallel.
Midpoint The midpoint of a line segment is the point that divides the segment into two equal parts.
Trapezoid Median Theorem The line segment joining the midpoints of the two parallel sides of a trapezoid is parallel to the other two sides and is equal to half the sum of their lengths.
Parallel Two lines are parallel if they lie in the same plane and never intersect.

View or post a solution

 

Discover Even More: See Also...

Problem 1520

Geometry Problem 1520: Discovering Distances in a Rectangle with an Exterior Point: A Geometry Challenge. Difficulty Level: High School.

Problem 1518

Geometry Problem 1518: Boost Your Geometry Skills: Solve for the Number of Sides in an Equiangular Polygon with an Interior Point and Bisected Angle. Difficulty Level: High School.

Problem 1517

Geometry Problem 1517: Unlocking Triangle Side Length: Solving with a Median and Two Angles. Difficulty Level: High School.

Problem 1516

Geometry Problem 1516: Finding the Length of a Side in an Equiangular Hexagon with Given Three Side Lengths

Problem 1515

Geometry Problem 1515: Mastering Triangle Distance Calculation: Find the Distance from the Intersection of Medians to an Exterior Line

Problem 1514

Geometry Problem 1514: Discover the Secret to Finding Distances in Regular Hexagons with Interior Squares. Difficulty Level: High School

Thematic Poem:
Geometry Unwound: The Power of Midpoints and Parallelogram

In a world of shapes and lines,
Where geometry defines,
A parallelogram stands tall,
With sides parallel to all.

Midpoints on the sides we see,
Where segments meet inevitably,
Drawing lines both straight and true,
Parallel to the sides they ensue.

At the intersection, a point appears,
The length of the segment now unclear,
But fear not, for math is here,
And with its power, the solution is near.

With formulas and calculations,
We find the length without hesitation,
Sum and divide, and solve with care,
And the length of the segment we declare.

Oh, the wonders of geometry,
And the power of discovery,
With midpoints and parallel lines,
The length of a segment unwinds.

So embrace the shapes and lines we see,
And let the beauty of math set us free,
For in this world of numbers and shapes,
The possibilities are vast, with no escape.

If you're interested in finding more poems with a focus on geometry, you may enjoy this collection: More geometry thematic poems.

Recent Additions

Geometry Problems
Open Problems
Visual Index
All Problems
Triangle
Midpoint
Parallel lines
Parallelogram
Trapezoid
Median of a Trapezoid
View or Post a solution

Search our site with Google: