Geometry Problem 1514: Discover the Secret to Finding Distances in Regular Hexagons with Interior Squares. Difficulty Level: High School.
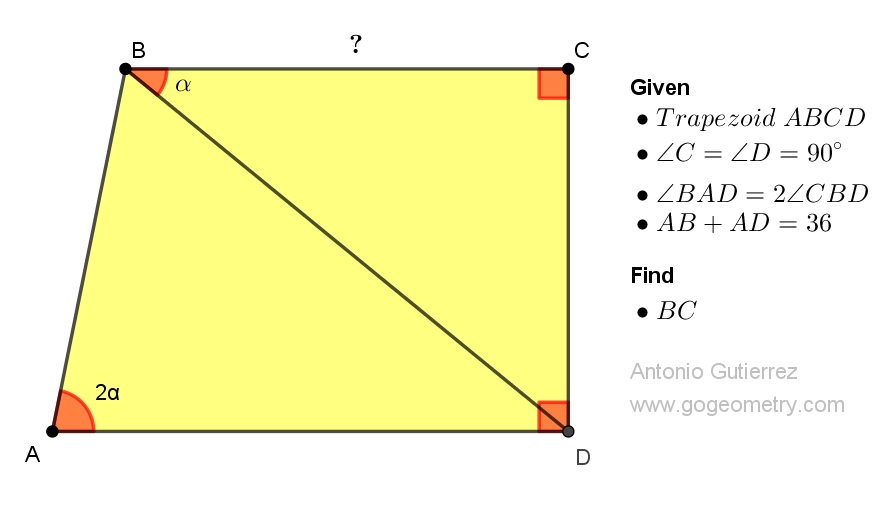
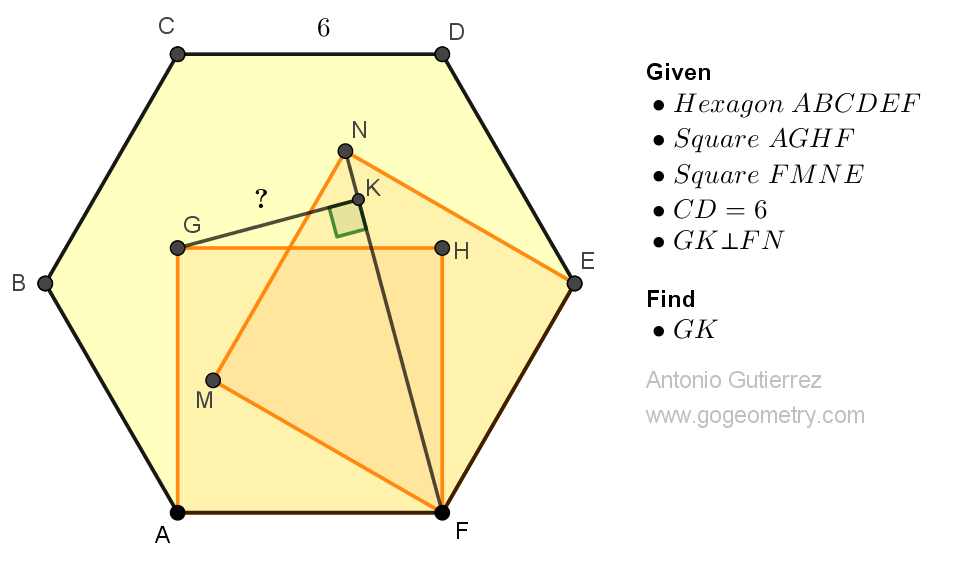
ABCDEF is a regular hexagon, and AGHF and FMNE are interior squares. If CD measures 6 units, calculate the distance from point G to line FN.
Definitions and Suggestions
- A regular hexagon is a six-sided polygon where all six sides have the same length and all six angles are the same size. Each internal angle of a regular hexagon measures 120 degrees, and the sum of all internal angles is 720 degrees.
- A square is a special type of rectangle where all four sides are of equal length and all four angles are right angles (90 degrees). Squares are commonly used in geometry and in real-world applications, such as in tiling, flooring, and building construction.
- The distance between a point and a line is the length of the perpendicular segment drawn from the point to the line. This distance is also called the shortest distance or perpendicular distance.
- Perpendicular lines are two lines that intersect at a right angle (90 degrees).
- A 30-60-90 triangle is a special type of right triangle with angles measuring 30 degrees, 60 degrees, and 90 degrees, respectively. The side opposite the 30-degree angle is half the length of the hypotenuse
Thematic Poem: Finding Distances in Regular Hexagons with Interior Squares
In a land of shapes and angles,
Where polygons reign supreme,
A
hexagon with squares inside,
Is quite a curious scene.
With
sides of equal length,
A perfect six-sided sight,
But to find the
distance from a point,
Will require some might.
For a vertex
must be measured,
To a side it must align,
A puzzle we must solve,
you see,
To find the answer fine.
With ruler, compass, and
our wits,
We'll measure and we'll draw,
And with each calculation
done,
We'll come one step closer to awe.
For the beauty of
geometry,
Is in its perfect form,
And in the solving of its
puzzles,
Our minds begin to transform.
So let us measure with
precision,
And let our hearts be true,
For in the land of shapes
and angles,
There's a world of wonder for me and you.