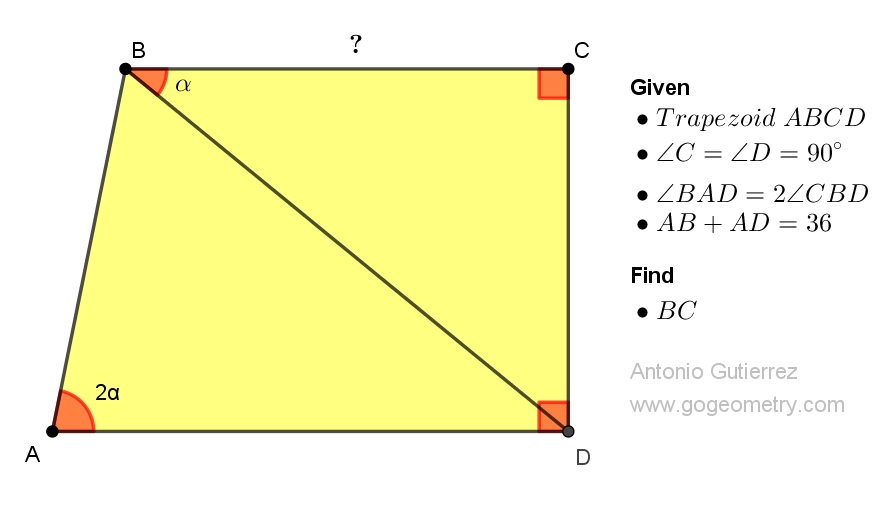
Geometry Problem 1513: Solving the base in a Right Trapezoid with Double Angle and Sum of Two Sides. Difficulty Level: High School.
In a right trapezoid ABCD, where angles C and D measure 90 degrees and angle BAD is twice angle CBD, if the sum of AB and AD is 36 units, calculate the measure of BC in units.
Definitions and Suggestions
- A triangle is a polygon that has three sides, three vertices, and three angles. Triangles are some of the most basic shapes in geometry and can be found in many different forms.
- A trapezoid, also known as a trapezium in British English, is a quadrilateral with at least one pair of parallel sides. The parallel sides are called the bases of the trapezoid, and the other two sides are called the legs.
- A right trapezoid, also known as a right trapezium, is a trapezoid with two adjacent right angles. In other words, one of its legs is perpendicular to both of its bases.
- An isosceles triangle is a type of triangle that has two sides of equal length and two angles opposite those sides of equal measure.
- In an isosceles triangle, the external double angle opposite to the base is the external angle at one of the vertices of the triangle that is opposite to the base.
- Two triangles are said to be congruent if all corresponding sides and angles of one triangle are equal to the corresponding sides and angles of the other triangle. There are several ways to prove that two triangles are congruent, including the Side-Side-Side (SSS), Side-Angle-Side (SAS), Angle-Side-Angle (ASA), and Hypotenuse-Leg (HL) criteria.
Thematic Poem: Solving the base in a Right Trapezoid with Double Angle and Sum of Two Sides
In a realm of shapes and lines
Geometry's secrets, it defines
A
right trapezoid, its sides known
But its base, a mystery, is shown
With a double angle to behold
And a sum of sides to be told
The
puzzle lies in its base
A solution we must embrace
Through calculation and deduction
We seek a geometric construction
Lines and angles we align
To unlock the answer, divine
With perseverance and wit
We solve the base, bit by bit
And
with newfound clarity and grace
We unveil geometry's hidden face
For in this world of shapes and math
Problems are but a simple
path
To unlock the secrets of the universe
And in our minds, a new
knowledge immerse.