Geometry Problem 1512: Finding the Length of a Segment in a Triangle with a Median and a Cevian with Given Ratio. Difficulty Level: High School.

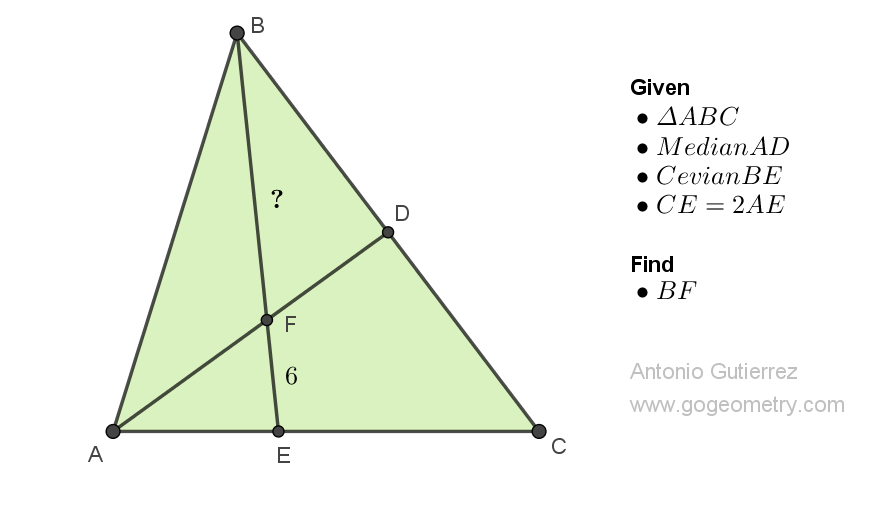
In a triangle ABC, AD is a median, and E is a point on AC such that CE = 2AE. AD and BE intersect at F. If EF measures 6 units, find the length of BF in units.

Geometry Problem 1512: Finding the Length of a Segment in a Triangle with a Median and a Cevian with Given Ratio. Difficulty Level: High School

Definitions and Suggestions

  • A triangle is a polygon that has three sides, three vertices, and three angles. Triangles are some of the most basic shapes in geometry and can be found in many different forms.
  • A median of a triangle is a line segment that connects a vertex of the triangle to the midpoint of the opposite side.
  • A cevian is a line segment that connects a vertex of a triangle to the opposite side (or its extension).
  • A given ratio of segments refers to the comparison of the lengths of two or more line segments in a specific ratio. Given ratios of segments can be used to solve problems related to scaling, similarity, and proportionality, among other things.
  • The Midsegment Theorem, also known as the Midline Theorem or the Base Midsegment Theorem states that the length of the midsegment of a triangle is equal to half the length of the third side of the triangle.

View or post a solution

 

Discover Even More: See Also...

Problem 1513

Geometry Problem 1513: Solving the base in a Right Trapezoid with Double Angle and Sum of Two Sides. Difficulty Level: High School

Problem 1511

Geometry Problem 1511: Finding the Altitude of an Isosceles Triangle Using Distances from a Point on the Extension of the Base. Difficulty Level: High School

Problem 1510

Geometry Problem 1510: Congruence of Triangles, Perimeter, Measurement. Difficulty Level: High School

Problem 1509

Geometry Problem 1509: Congruence of Triangles in a Trapezoid and a Square, Measurement. Difficulty Level: High School

Problem 1508

Geometry Problem 1508: Understanding Triangle Geometry: Isosceles, Equilateral, Quadrilateral, Angular. Difficulty Level: High School

Problem 1507

Geometry Problem 1507: Understanding Triangle Geometry: Double Angles, Altitudes, and Measurements. Difficulty Level: High School

Thematic Poem: The Triumph of Reason: Solving Geometry Problems with Logic and Perseverance

In geometry class, we learn of shapes
And how to measure all their gapes
Triangles, with angles three
And sides to calculate, oh gee!

A problem posed, with median and cevian
To find the segment's length, we're cravin'
With a given ratio of one to two
Our minds race with what to do

With ruler and compass, we draw
The lines we need, without a flaw
Then use the theorems we've been taught
To find the length, as we ought

We calculate and solve the equation
Our hearts race with anticipation
And finally, we reach the solution
A feeling of pride, a grand conclusion

In geometry, we find our way
Using logic and reason every day
And though the problems may be tough
We persevere, and that's enough.

If you're interested in finding more poems with a focus on geometry, you may enjoy this collection: More geometry thematic poems.

Recent Additions

Geometry Problems
Open Problems
Visual Index
All Problems
Triangle
Median
Similarity, Ratios, Proportions
Midpoints
View or Post a solution

Search our site with Google: