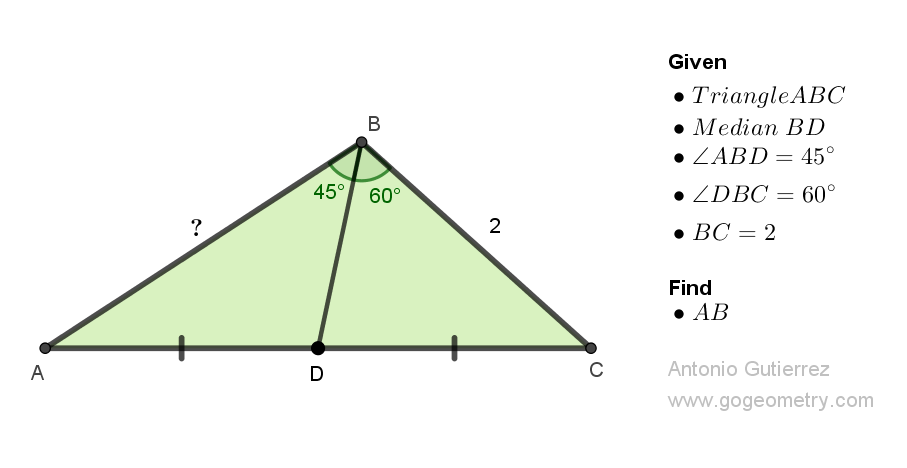
Geometry Problem 1517: Unlocking Triangle Side Length: Solving with a Median and Two Angles. Difficulty Level: High School.

In triangle ABC, BD is a median, and angles ABD and DBC measure 45 and 60 degrees, respectively. If BC measures 2, calculate the length of AB.

Geometry Problem 1517: Unlocking Triangle Side Length: Solving with a Median and Two Angles. Difficulty Level: High School.

Definitions and Suggestions

Geometric Element Theorem Statement
30-60-90 Triangle Theorem In a 30-60-90 triangle, the length of the hypotenuse is twice the length of the shorter leg, and the length of the longer leg is the square root of 3 times the length of the shorter leg.
45-45-90 Triangle Theorem In a 45-45-90 triangle, the length of the hypotenuse is the square root of 2 times the length of either leg.
Median Definition In a triangle, the median from a vertex divides the opposite side into two segments of equal length.
Midsegment Theorem In a triangle, the midsegment connecting the midpoints of two sides is parallel to the third side and half its length.

View or post a solution

 

Discover Even More: See Also...

Problem 1518

Geometry Problem 1518: Boost Your Geometry Skills: Solve for the Number of Sides in an Equiangular Polygon with an Interior Point and Bisected Angle. Difficulty Level: High School.

Problem 1516

Geometry Problem 1516: Finding the Length of a Side in an Equiangular Hexagon with Given Three Side Lengths

Problem 1515

Geometry Problem 1515: Mastering Triangle Distance Calculation: Find the Distance from the Intersection of Medians to an Exterior Line

Problem 1514

Geometry Problem 1514: Discover the Secret to Finding Distances in Regular Hexagons with Interior Squares. Difficulty Level: High School

Problem 1513

Geometry Problem 1513: Solving the base in a Right Trapezoid with Double Angle and Sum of Two Sides. Difficulty Level: High School

Problem 1512

Geometry Problem 1512: Finding the Length of a Segment in a Triangle with a Median and a Cevian with Given Ratio. Difficulty Level: High School

Thematic Poem:
Unlocking Triangle Side Length with a Median and Two Angles

Unlocking triangle side length
A puzzle that seems to have no end
But with a median and two angles to tend
The solution we can apprehend.

The median divides the triangle in two
And gives us angles to eschew
With symmetry as our cue
We can find the missing sides anew.

Geometry comes to our aid
To solve for the length of the blade
And with the angles that we've made
The answer is found, no need to persuade.

Unlocking triangle side length
With a median and angles to represent
We see the beauty of math's extent
And the solutions it can present.

If you're interested in finding more poems with a focus on geometry, you may enjoy this collection: More geometry thematic poems.

Recent Additions

Geometry Problems
Open Problems
Visual Index
All Problems
Triangle
Median
Midpoint
45-45-90 degrees
30-60-90 degrees
Parallel lines
View or Post a solution

Search our site with Google: