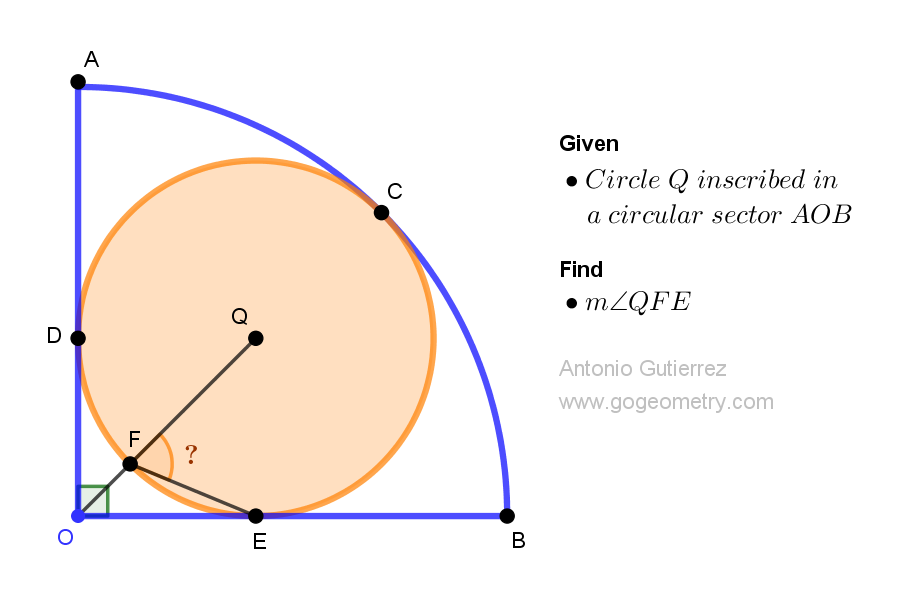
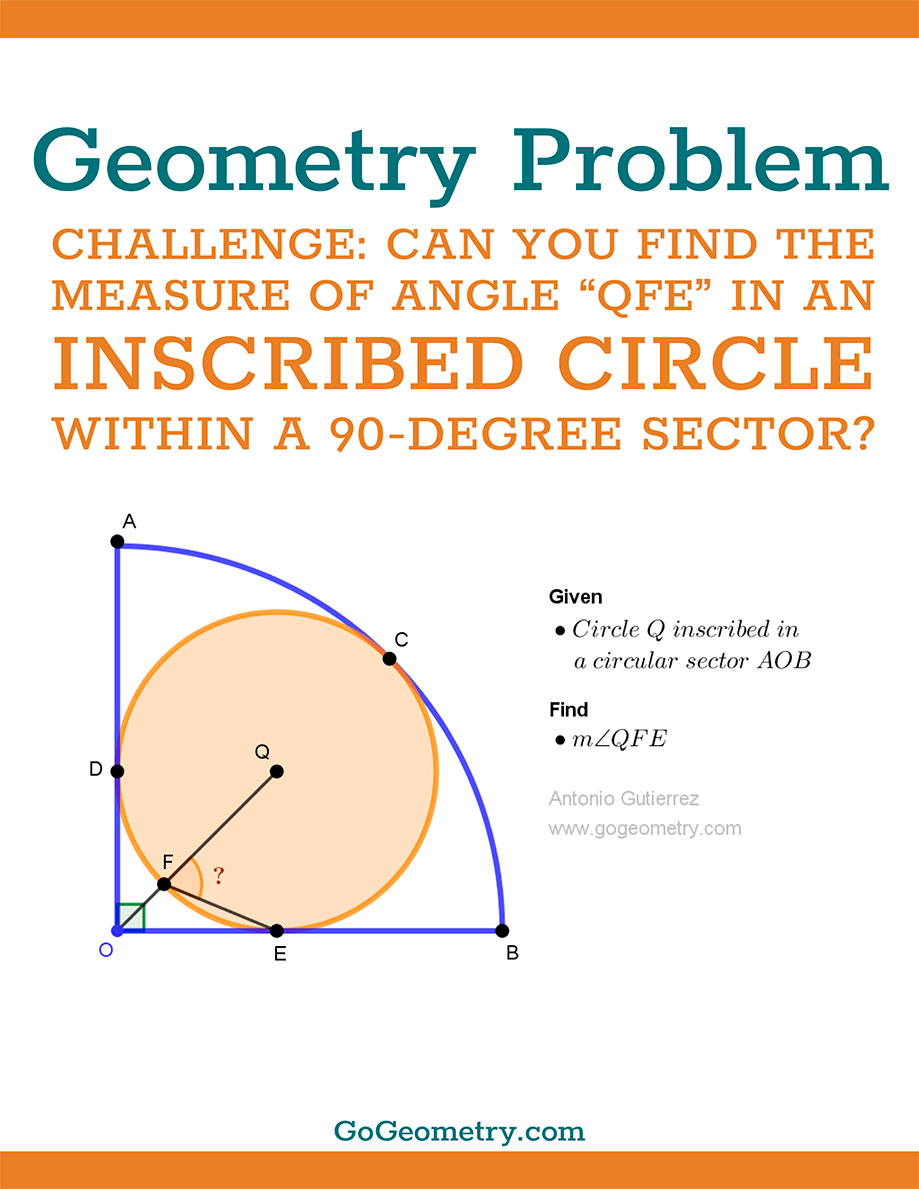
Geometry Problem 1528: Cracking the Circle Code: Unveiling the Tangent and Angle of an Inscribed Circle within a 90-Degree Circular Sector. Difficulty Level: High School.
A circle with center Q is inscribed in a circular sector AOB with a 90-degree angle and center O. The circle is tangent to OB at point E. Find the measure of angle QFE.
View or post a solution
Flyer of problem 1528 using iPad Apps
Discover Even More: See Also...
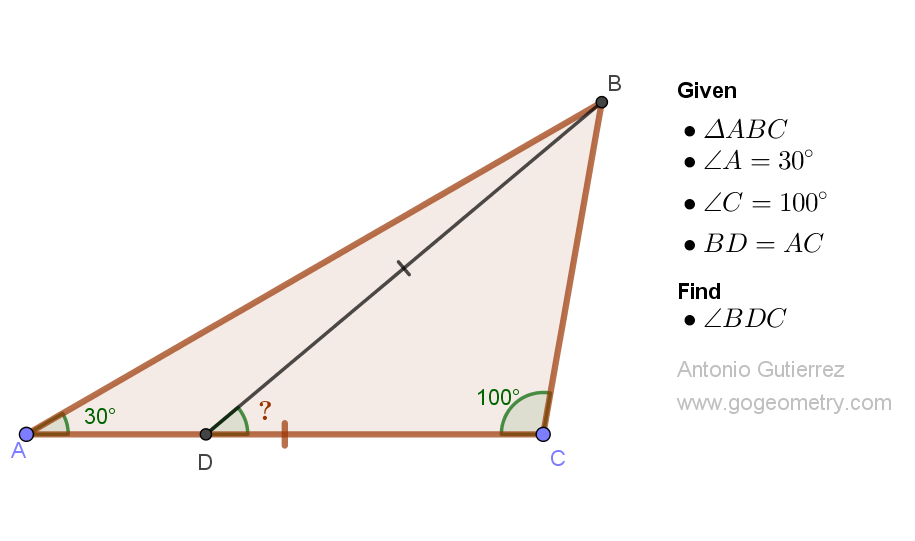
Problem 1529
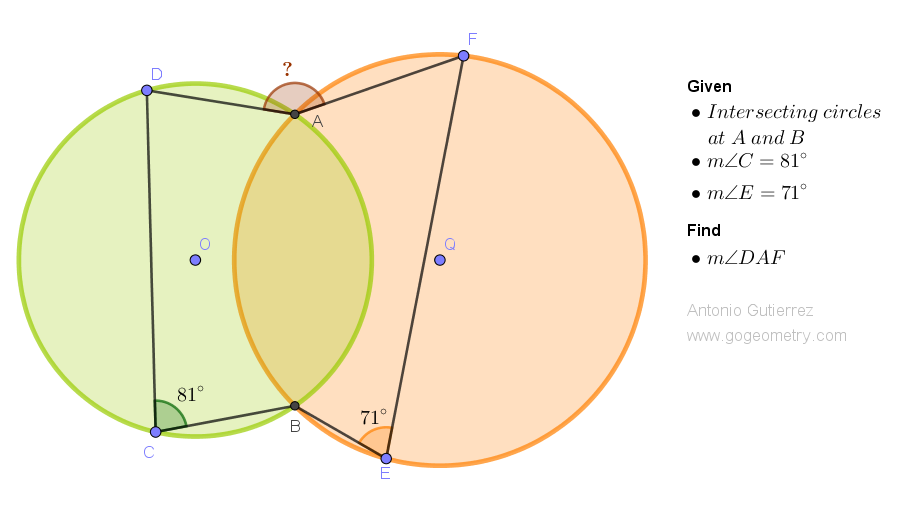
Problem 1527
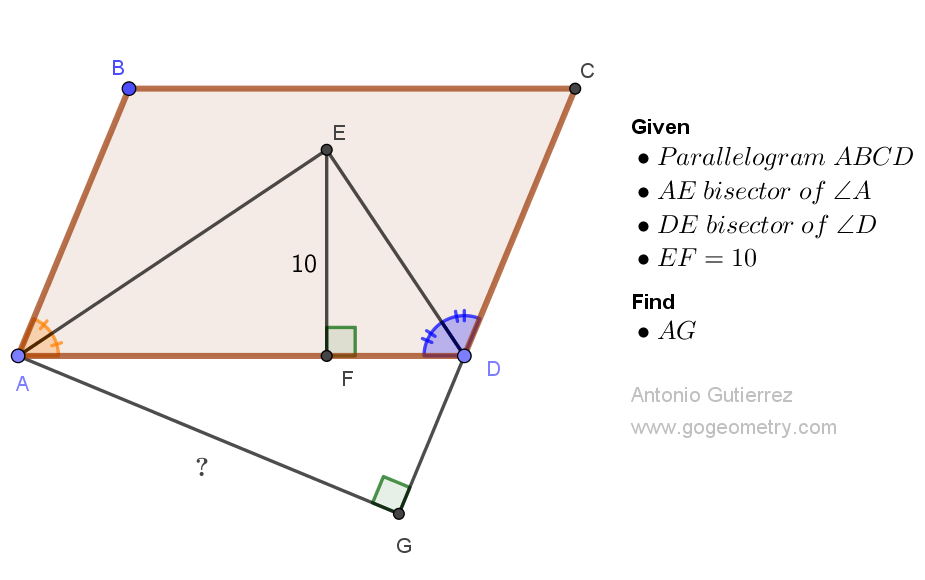
Problem 1526
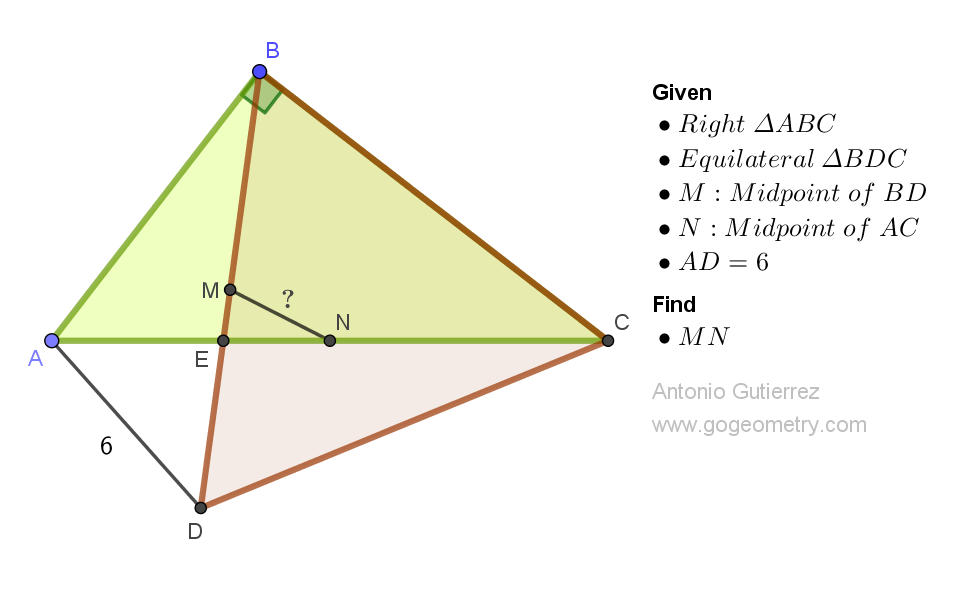
Problem 1525
Problem 1524
Problem 1523
Thematic Poem:
Unlocking the Circle's Hidden Code
Within a circle's bounds we seek,
To find the
hidden code unique.
A tangent and an angle rare,
Within a sector, we'll find them there.
With careful measure and calculation,
We'll unlock
this circle's foundation.
For in its inscribed form
we see,
A world of geometry.
The tangent line, it touches close,
A point of
contact we disclose.
And from that point, we measure
true,
The angle that we seek anew.
Within this ninety-degree space,
We find the
circle's hidden grace.
Its tangents and angles now
revealed,
A treasure trove that's unconcealed.
If you're interested in finding more poems with a focus on geometry, you may enjoy this collection: More geometry thematic poems.
Recent Additions
Geometry Problems
Open Problems
Visual Index
All Problems
Circle
Circular Sector
Angle
Circle Tangent Line
Perpendicular lines
Isosceles Triangle
View or Post a solution
Search our site with Google: