Math Education Geometry Problem 871: Brahmagupta's Theorem, Cyclic Quadrilateral, Perpendicular Diagonals, Midpoint. Level: School, College, Mathematics Education. Distance learning.

Online Geometry Problem 871: Brahmagupta's Theorem, Cyclic Quadrilateral, Perpendicular Diagonals, Midpoint. Level: High School, College, Mathematics Education

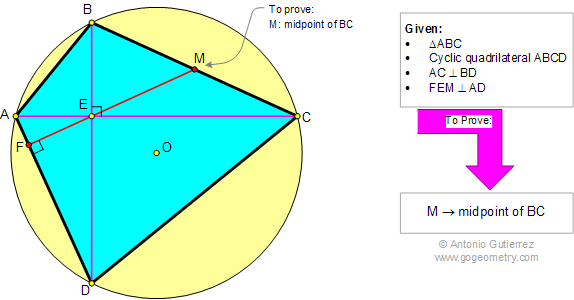
Brahmagupta's theorem states that if a cyclic quadrilateral ABCD has perpendicular diagonals, then the perpendicular to a side from the point of intersection of the diagonals always bisects the opposite side.

Brahmagupta's Theorem, Cyclic Quadrilateral, Perpendicular Diagonals, Midpoint

Brahmagupta (598–668) was an Indian mathematician and astronomer who discovered a neat formula for the area of a cyclic quadrilateral.

See also: Corollary, Area Formula, Area Formula Extension, .

Geometry problem solving is one of the most challenging skills for students to learn. When a problem requires auxiliary construction, the difficulty of the problem increases drastically, perhaps because deciding which construction to make is an ill-structured problem. By “construction,” we mean adding geometric figures (points, lines, planes) to a problem figure that wasn’t mentioned as "given."