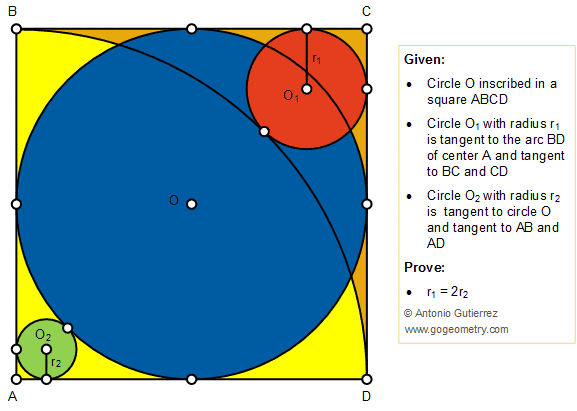
A circle O is inscribed in a square ABCD. As shown in the figure below, a circle O₁ with radius r₁ is tangent to the arc BD of center A and tangent to BC and CD. A circle O₂ with radius r₂ is tangent to circle O and tangent to AB and AD. Prove that r₁ = 2r₂.
 

See solution below

---

Geometric Art of Problem 1427: Sketching, Typography, iPad Apps

---

Animation of the Conformal Mapping or Transformation of Problem 1427

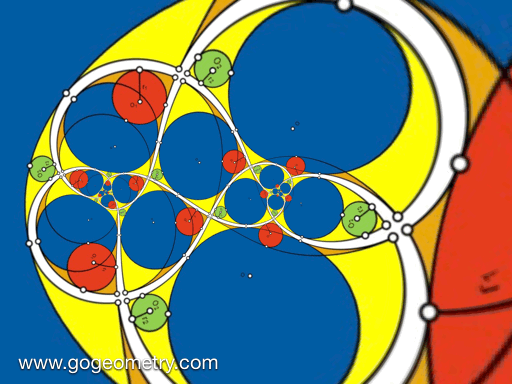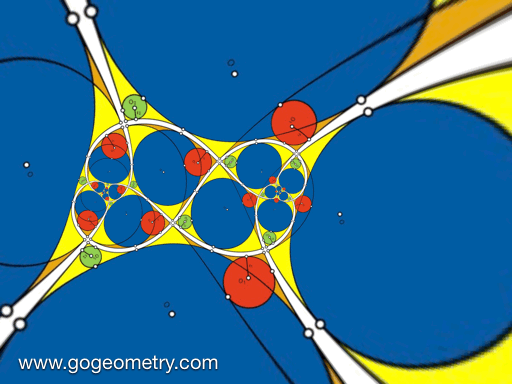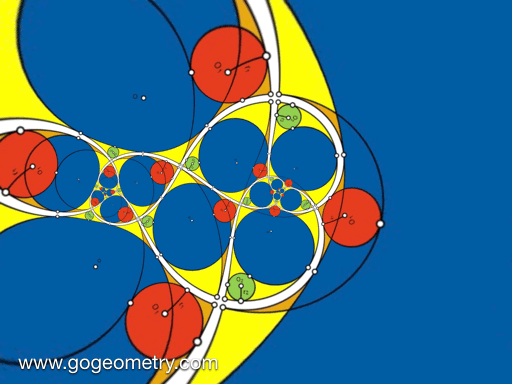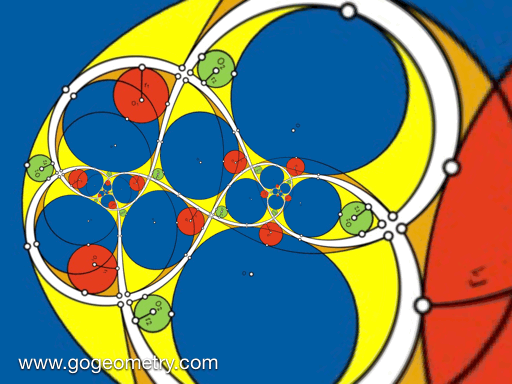
Conformal Mapping

A conformal mapping or conformal transformation is a continuous mapping preserving the form of infinitesimal figures. This conformal map produces a realistic view of the original image or map. This the conformal transformation of problem1427 

See also: Typography and poster of problem 1426.