Garden Geometry 1585: The Hidden Harmony of Paths and Tangent Points

Discover how a triangle's incircle reveals the secret of harmonious convergence.

In this exploration, you'll dive into the elegant interplay of tangency points, pathways, and concurrency - perfect for designing gardens or understanding deeper mathematical truths.

Problem 1585 Statement

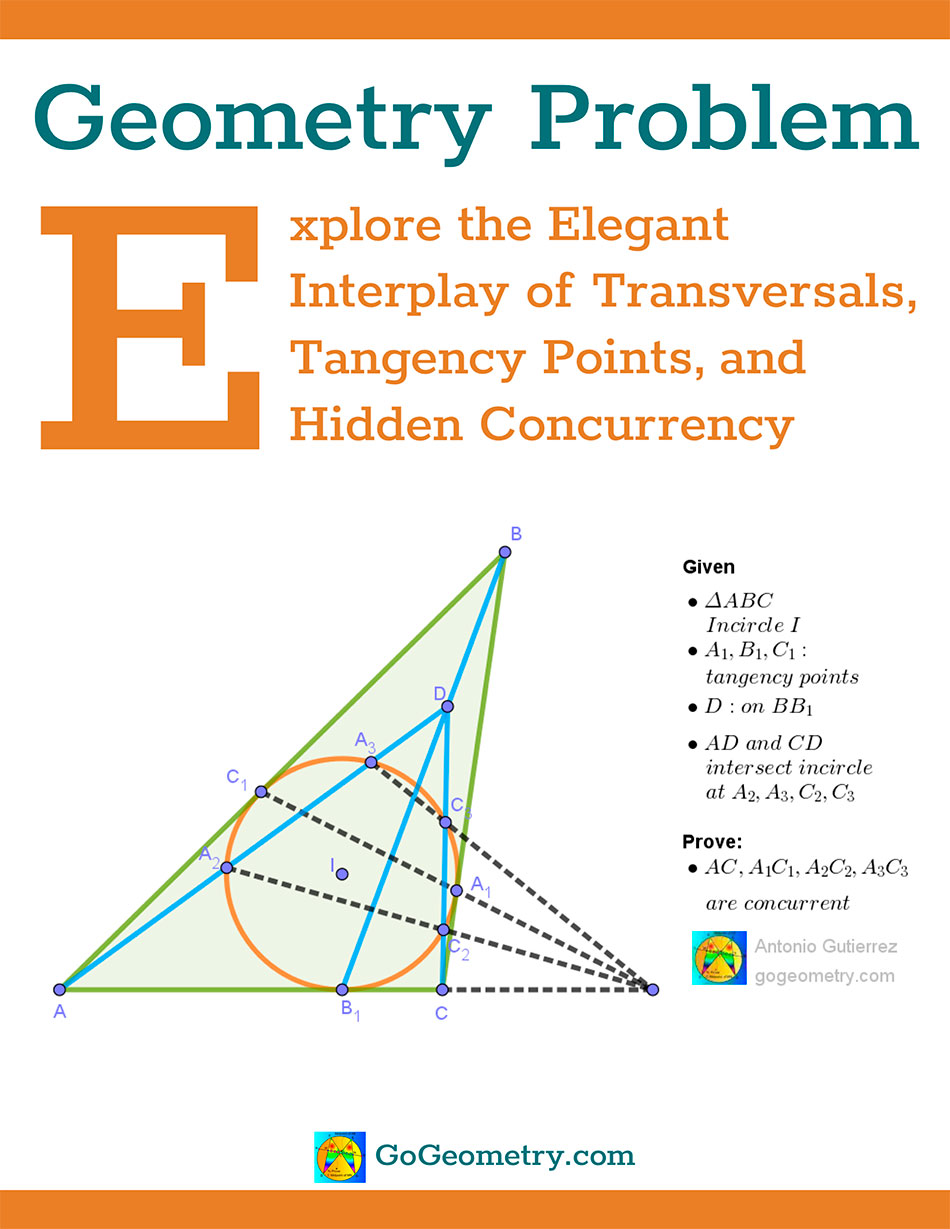
The incircle of triangle ABC is tangent to sides BC, AC, and AB at points A₁, B₁, and C₁, respectively. If D is an arbitrary point on segment BB₁ and lines AD and CD intersect the incircle at points A₂, A₃, and C₂, C₃, respectively, prove that lines AC, A₁C₁, A₂C₂, and A₃C₃ are concurrent.

Uncover and share solutions to this problem.

---

Flyer of problem 1585 Designed with iPad Apps