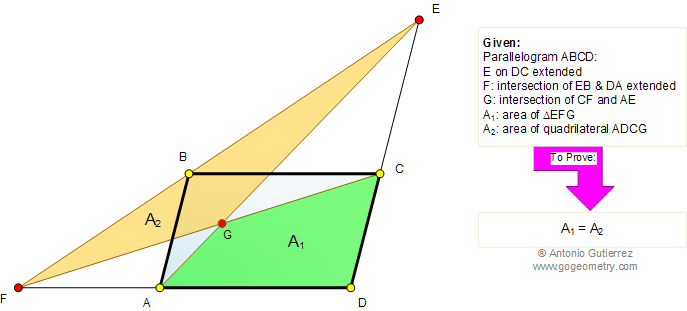
Geometry Problem 489. Proving the Equality of Areas in a Parallelogram and Extended Lines Intersection: Parallelogram, Triangle, Quadrilateral, Area. Level: High School, College, SAT Prep.

The figure shows a parallelogram ABCD, with point E located on the extended line of DC. The extended line of EB intersects the extended line of DA at point F. Lines CF and AE meet at point G. Prove that the area of triangle EFG is equal to the area of quadrilateral ADCG

 

Thematic poem of Problem 489

In the realm of shapes and lines,
Geometry's beauty surely shines.
With problem 489, we are tasked,
To prove an equality that's masked.

A parallelogram, with sides so true,
Stands strong in its quadrilateral view.
Extended lines and points that meet,
Make the problem oh so sweet.

Triangle EFG, with angles bold,
Is the shape that we must behold.
And quadrilateral ADCG we see,
Is where the proof must truly be.

Area equality is the aim,
To solve this problem with no shame.
Geometry's tools we must employ,
And the proof we'll surely enjoy.

With logic, reason, and a bit of grace,
The solution slowly takes its place.
Parallelogram, triangle, quadrilateral, area,
All work together in perfect criteria.

And as we solve this problem so grand,
We see geometry's beauty firsthand.
Shapes and lines that we can't deny,
Are truly captivating to the eye.