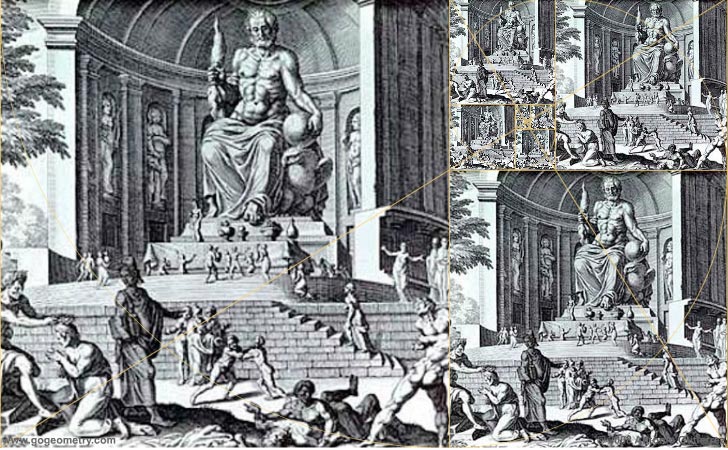
The Statue of Zeus at Olympia from a drawing by Maarten van Heemskerck

Successive Golden Rectangles dividing a Golden Rectangle into squares (An engraving of the Statue of Zeus made by Philippe Galle in 1572).

Zeus, king of the gods,
His statue a wonder stood,
Olympia's grand feat.

A golden rectangle is a rectangle whose side lengths are in the golden ratio, one-to-phi, that is, approximately 1:1.618. A distinctive feature of this shape is that when a square section is removed, the remainder is another golden rectangle, that is, with the same proportions as the first. Square removal can be repeated infinitely, which leads to an approximation of the golden or Fibonacci spiral.

Fibonacci numbers (0,1,1,2,3,5,8,13,21,34...) are a sequence of numbers named after Leonardo of Pisa, known as Fibonacci. The first number of the sequence is 0, the second number is 1, and each subsequent number is equal to the sum of the previous two numbers of the sequence itself.

The Statue of Zeus at Olympia was one of the classic Seven Wonders of the Ancient World. It was made by the famed Greek sculptor of the Classical period, Phidias, circa 432 BC on the site where it was erected in the temple of Zeus, Olympia, Greece. Source: Wikipedia: Statue of Zeus at Olympia.